what is given in problem solving in math

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Free ready-to-use math resources

Hundreds of free math resources created by experienced math teachers to save time, build engagement and accelerate growth

20 Effective Math Strategies To Approach Problem-Solving 

Katie Keeton

Math strategies for problem-solving help students use a range of approaches to solve many different types of problems. It involves identifying the problem and carrying out a plan of action to find the answer to mathematical problems.  

Problem-solving skills are essential to math in the general classroom and real-life. They require logical reasoning and critical thinking skills. Students must be equipped with strategies to help them find solutions to problems.

This article explores mathematical problem solving strategies, logical reasoning and critical thinking skills to help learners with solving math word problems independently in real-life situations. 

What are problem-solving strategies?

Problem-solving strategies in math are methods students can use to figure out solutions to math problems. Some problem-solving strategies: 

• Draw a model
• Use different approaches
• Check the inverse to make sure the answer is correct

Students need to have a toolkit of math problem-solving strategies at their disposal to provide different ways to approach math problems. This makes it easier to find solutions and understand math better. 

Strategies can help guide students to the solution when it is difficult ot know when to start.

The ultimate guide to problem solving techniques

Download these ready-to-go problem solving techniques that every student should know. Includes printable tasks for students including challenges, short explanations for teachers with questioning prompts.

20 Math Strategies For Problem-Solving

Different problem-solving math strategies are required for different parts of the problem. It is unlikely that students will use the same strategy to understand and solve the problem. 

Here are 20 strategies to help students develop their problem-solving skills. 

Strategies to understand the problem

Strategies that help students understand the problem before solving it helps ensure they understand: 

• The context
• What the key information is
• How to form a plan to solve it

Following these steps leads students to the correct solution and makes the math word problem easier .

Here are five strategies to help students understand the content of the problem and identify key information. 

1. Read the problem aloud

Read a word problem aloud to help understand it. Hearing the words engages auditory processing. This can make it easier to process and comprehend the context of the situation.

2. Highlight keywords 

When keywords are highlighted in a word problem, it helps the student focus on the essential information needed to solve it. Some important keywords help determine which operation is needed.  For example, if the word problem asks how many are left, the problem likely requires subtraction.  Ensure students highlight the keywords carefully and do not highlight every number or keyword. There is likely irrelevant information in the word problem.

3. Summarize the information

Read the problem aloud, highlight the key information and then summarize the information. Students can do this in their heads or write down a quick summary.  Summaries should include only the important information and be in simple terms that help contextualize the problem.

4. Determine the unknown

A common problem that students have when solving a word problem is misunderstanding what they are solving. Determine what the unknown information is before finding the answer.  Often, a word problem contains a question where you can find the unknown information you need to solve. For example, in the question ‘How many apples are left?’ students need to find the number of apples left over.

5. Make a plan

Once students understand the context of the word problem, have dentified the important information and determined the unknown, they can make a plan to solve it.  The plan will depend on the type of problem. Some problems involve more than one step to solve them as some require more than one answer.  Encourage students to make a list of each step they need to take to solve the problem before getting started.

Strategies for solving the problem 

1. draw a model or diagram.

Students may find it useful to draw a model, picture, diagram, or other visual aid to help with the problem solving process.  It can help to visualize the problem to understand the relationships between the numbers in the problem. In turn, this helps students see the solution.

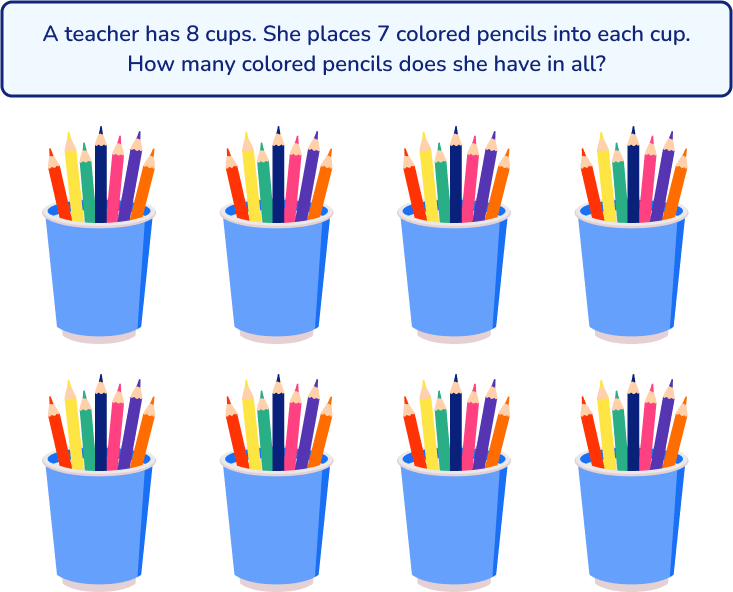
Similarly, you could draw a model to represent the objects in the problem:

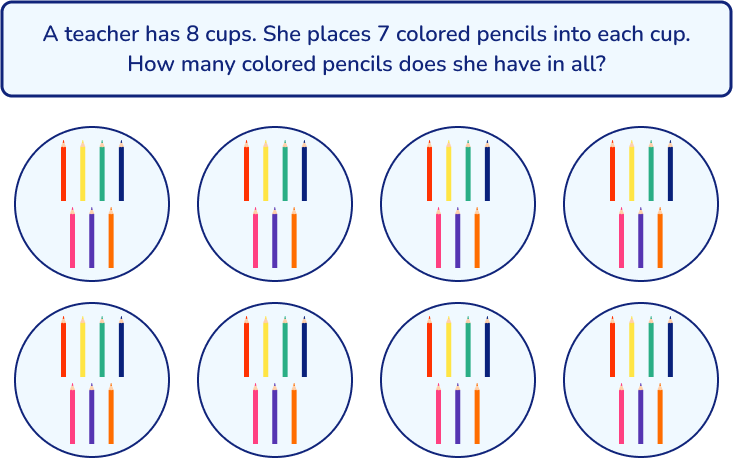
2. Act it out

This particular strategy is applicable at any grade level but is especially helpful in math investigation in elementary school . It involves a physical demonstration or students acting out the problem using movements, concrete resources and math manipulatives .  When students act out a problem, they can visualize and contectualize the word problem in another way and secure an understanding of the math concepts.  The examples below show how 1st-grade students could “act out” an addition and subtraction problem:

3. Work backwards

Working backwards is a popular problem-solving strategy. It involves starting with a possible solution and deciding what steps to take to arrive at that solution.  This strategy can be particularly helpful when students solve math word problems involving multiple steps. They can start at the end and think carefully about each step taken as opposed to jumping to the end of the problem and missing steps in between.

For example,

To solve this problem working backwards, start with the final condition, which is Sam’s grandmother’s age (71) and work backwards to find Sam’s age. Subtract 20 from the grandmother’s age, which is 71.  Then, divide the result by 3 to get Sam’s age. 71 – 20 = 51 51 ÷ 3 = 17 Sam is 17 years old.

4. Write a number sentence

When faced with a word problem, encourage students to write a number sentence based on the information. This helps translate the information in the word problem into a math equation or expression, which is more easily solved.  It is important to fully understand the context of the word problem and what students need to solve before writing an equation to represent it.

5. Use a formula

Specific formulas help solve many math problems. For example, if a problem asks students to find the area of a rug, they would use the area formula (area = length × width) to solve.   Make sure students know the important mathematical formulas they will need in tests and real-life. It can help to display these around the classroom or, for those who need more support, on students’ desks.

Strategies for checking the solution 

Once the problem is solved using an appropriate strategy, it is equally important to check the solution to ensure it is correct and makes sense. 

There are many strategies to check the solution. The strategy for a specific problem is dependent on the problem type and math content involved.

Here are five strategies to help students check their solutions. 

1. Use the Inverse Operation

For simpler problems, a quick and easy problem solving strategy is to use the inverse operation. For example, if the operation to solve a word problem is 56 ÷ 8 = 7 students can check the answer is correct by multiplying 8 × 7. As good practice, encourage students to use the inverse operation routinely to check their work. 

2. Estimate to check for reasonableness

Once students reach an answer, they can use estimation or rounding to see if the answer is reasonable.  Round each number in the equation to a number that’s close and easy to work with, usually a multiple of ten.  For example, if the question was 216 ÷ 18 and the quotient was 12, students might round 216 to 200 and round 18 to 20. Then use mental math to solve 200 ÷ 20, which is 10.  When the estimate is clear the two numbers are close. This means your answer is reasonable. 

3. Plug-In Method

This method is particularly useful for algebraic equations. Specifically when working with variables.  To use the plug-in method, students solve the problem as asked and arrive at an answer. They can then plug the answer into the original equation to see if it works. If it does, the answer is correct.

If students use the equation 20m+80=300 to solve this problem and find that m = 11, they can plug that value back into the equation to see if it is correct. 20m + 80 = 300 20 (11) + 80 = 300 220 + 80 = 300 300 = 300 ✓

4. Peer Review

Peer review is a great tool to use at any grade level as it promotes critical thinking and collaboration between students. The reviewers can look at the problem from a different view as they check to see if the problem was solved correctly.   Problem solvers receive immediate feedback and the opportunity to discuss their thinking with their peers. This strategy is effective with mixed-ability partners or similar-ability partners. In mixed-ability groups, the partner with stronger skills provides guidance and support to the partner with weaker skills, while reinforcing their own understanding of the content and communication skills.  If partners have comparable ability levels and problem-solving skills, they may find that they approach problems differently or have unique insights to offer each other about the problem-solving process.

5. Use a Calculator

A calculator can be introduced at any grade level but may be best for older students who already have a foundational understanding of basic math operations. Provide students with a calculator to allow them to check their solutions independently, accurately, and quickly. Since calculators are so readily available on smartphones and tablets, they allow students to develop practical skills that apply to real-world situations.  

Step-by-step problem-solving processes for your classroom

In his book, How to Solve It , published in 1945, mathematician George Polya introduced a 4-step process to solve problems. 

Polya’s 4 steps include:

• Understand the problem
• Devise a plan
• Carry out the plan

Today, in the style of George Polya, many problem-solving strategies use various acronyms and steps to help students recall. 

Many teachers create posters and anchor charts of their chosen process to display in their classrooms. They can be implemented in any elementary, middle school or high school classroom. 

Here are 5 problem-solving strategies to introduce to students and use in the classroom.

How Third Space Learning improves problem-solving 

Resources .

Third Space Learning offers a free resource library is filled with hundreds of high-quality resources. A team of experienced math experts carefully created each resource to develop students mental arithmetic, problem solving and critical thinking. 

Explore the range of problem solving resources for 2nd to 8th grade students. 

One-on-one tutoring 

Third Space Learning offers one-on-one math tutoring to help students improve their math skills. Highly qualified tutors deliver high-quality lessons aligned to state standards. 

Former teachers and math experts write all of Third Space Learning’s tutoring lessons. Expertly designed lessons follow a “my turn, follow me, your turn” pedagogy to help students move from guided instruction and problem-solving to independent practice. 

Throughout each lesson, tutors ask higher-level thinking questions to promote critical thinking and ensure students are developing a deep understanding of the content and problem-solving skills.

Problem-solving

Educators can use many different strategies to teach problem-solving and help students develop and carry out a plan when solving math problems. Incorporate these math strategies into any math program and use them with a variety of math concepts, from whole numbers and fractions to algebra. 

Teaching students how to choose and implement problem-solving strategies helps them develop mathematical reasoning skills and critical thinking they can apply to real-life problem-solving.

READ MORE :

• 8 Common Core math examples
• Tier 3 Interventions: A School Leaders Guide
• Tier 2 Interventions: A School Leaders Guide
• Tier 1 Interventions: A School Leaders Guide

There are many different strategies for problem-solving; Here are 5 problem-solving strategies: • draw a model  • act it out  • work backwards  • write a number sentence • use a formula

Here are 10 strategies for problem-solving: • Read the problem aloud • Highlight keywords • Summarize the information • Determine the unknown • Make a plan • Draw a model  • Act it out  • Work backwards  • Write a number sentence • Use a formula

1. Understand the problem 2. Devise a plan 3. Carry out the plan 4. Look back

Some strategies you can use to solve challenging math problems are: breaking the problem into smaller parts, using diagrams or models, applying logical reasoning, and trying different approaches.

Related articles

How To Teach Math: 10 Effective Strategies For Teaching Math In The Classroom

Building Thinking Classrooms: Effective Strategies For Your Math Classroom

Differentiation Strategies In The Classroom: 8 Methods For Every Teacher

Retrieval Practice: A Foolproof Method To Improve Student Retention and Recall

Ultimate Guide to Metacognition [FREE]

Looking for a summary on metacognition in relation to math teaching and learning?

Check out this guide featuring practical examples, tips and strategies to successfully embed metacognition across your school to accelerate math growth.

Privacy Overview

• Math Tutorial

What Is Problem Solving In Mathematics?

Definition and importance of problem solving in mathematics

Problem solving in mathematics is more than just crunching numbers and arriving at an answer. It’s a fundamental part of the subject that encourages you to:

• Understand the problem : Grasp what is being asked. What are the unknowns, and what information do you have?
• Develop reasoning skills : Going beyond memorization, problem solving involves logical thinking and the ability to reason out various solutions.
• Apply knowledge creatively : Use what you know in new and varied contexts.
• Overcome challenges : Develop perseverance, as often the first approach may not lead to a solution.

This component of mathematics helps you in real-life situations because life , much like math, is full of problems waiting to be solved. It’s about being adaptable, methodical, and open-minded.

Key skills and strategies for effective problem solving

To master problem solving, certain skills and strategies are essential, including:

• Analytical thinking : Break down complex problems into manageable parts.
• Pattern recognition : Notice recurring themes that can simplify the process.
• Communication : Be able to articulate the problem and explain your solution to others.
• Critical thinking : Evaluate and improve your approach.

Some tried-and-true strategies that aid in problem solving are:

• Working backwards : Start from the desired outcome and trace steps back to the starting point.
• Making a list  or  table : Organize information systematically.
• Drawing a diagram or picture : Visual aids can make abstract problems more concrete.
• Guessing and checking : Make educated guesses and refine them based on feedback.
• Looking for analogies : Compare with problems you’ve already solved.

Remember, with practice and patience, you can strengthen your problem-solving muscles in mathematics and beyond. Each puzzle you encounter is a chance to learn and grow, so embrace the challenges!

Understanding the Problem

Read and analyze the problem statement.

When facing a new mathematical challenge, the first step is always to carefully read and analyze the problem statement. Don’t rush through this; take your time to fully grasp what the problem is about. It’s like being a detective – pay attention to every detail, as sometimes even a single word can alter the meaning of a problem. This careful reading sets the groundwork for your entire problem-solving approach.

• Look for key information : Pay close attention to facts, figures, and the question.
• Clarify the goals : Determine what the problem is asking you to find.
• Take notes : Write down important details that might help you later.

Identify the known and unknown quantities

Separating what you know from what you don’t know is like sorting puzzle pieces by color before you start assembling the picture. It’s a vital part of the process that gives you clear direction on where to focus your efforts.

• List what’s given : Jot down all the information provided in the problem.
• Pinpoint what’s missing : Recognize what you’re trying to find.
• Make assumptions, if necessary : Sometimes, you need to fill in gaps with logical guesses.

Remember, everyone makes mistakes, and that’s okay. Embrace them as learning opportunities. You’ve got this! By taking these initial steps, you’re laying a solid foundation to tackle mathematical problems with confidence. So, keep practicing, stay curious, and remind yourself that with each problem a little bit of persistence goes a long way. Good luck – you’re on your way to becoming a math problem-solving wizard!

Problem Solving Approaches

Trial and error method.

Have you ever heard of the trial and error method? It’s like trying on shoes until you find the perfect fit. Here’s how you can apply this technique to your math problems:

Experiment with different techniques: There’s more than one way to solve a problem and it’s totally okay to try them out until one sticks.

Learn from mistakes: Each error is a step closer to understanding what doesn’t work, narrowing down the right path for you.

Be patient and persistent: Stick with it, even if you don’t get it on the first try. Perseverance is your best friend in this case.

Remember, there’s no shame in stumbling along the way; it’s all part of the learning curve. You’re doing great by just giving it a shot!

Using a Systematic Approach

Systematic approaches are a bit like following a recipe. You have a step-by-step guide that helps you stay organized and focused. Try these strategies:

Outline a plan: Sketch out the steps you need to take to arrive at the solution. A plan can steer you in the right direction.

Break problems into smaller parts: Don’t overwhelm yourself; slice that bigger problem into manageable pieces.

Validate each step: As you work through the problem, check each part to make sure you’re on track.

Using a systematic approach can make complex problems feel more manageable, and before you know it, you’ll have arrived at the answer without feeling lost or frustrated. Keep going—you’ve got the tools you need to crack even the toughest problems!

Problem Solving Strategies

Working backwards method.

Sometimes the best way to tackle a problem is to start at the end and work your way back to the beginning. Here’s why this can be a game-changer for you:

Reverse-engineering the solution: If you know where you need to end up, try figuring out the steps that led there—it’s like solving a mystery backward!

Simplifies complex problems: Working backwards can often simplify the process since the endpoint is your guide.

Highlights necessary information: This method helps you identify the most crucial pieces of information needed to solve the problem.

Give this approach a try next time you’re confronted with a particularly tricky puzzle. Who knows, you may just uncover a shortcut to the solution!

Making a Table or Chart

Are you a visual learner? Making tables or charts can be particularly effective for you. They help organize information and can highlight patterns or relationships that aren’t immediately obvious. Consider the following advantages of this strategy:

Visual organization: A table or chart can make complex data more digestible and approachable.

Track progress: Seeing your data laid out can help you track where you are in the problem-solving process.

Identify patterns: Sometimes the key to solving a problem lies in a pattern that only becomes visible when data is laid out visually.

So, next time you feel overwhelmed by a bunch of numbers or data points, take a step back and try putting them into a chart or table. It might just provide the clarity you need to crack the code! Keep pushing forward; your problem-solving toolkit is getting more robust by the minute.

Problem Solving Techniques

Using diagrams or visual representations.

I have another neat trick up my sleeve to share with you, and it’s all about getting visual with diagrams! Here’s why you should consider drawing your way out of a problem:

Makes abstract ideas concrete: Sometimes, issues feel tangled and chaotic in your mind. Sketching them out can make the abstract tangible. 

Engages different parts of the brain: Using visuals can engage your brain’s right hemisphere, which is thought to be responsible for creativity and visualization.

Facilitates communication: When you’re working in a team, a diagram can be a universal language, making it easier for everyone to understand the issue at hand.

Go ahead, grab that pencil and start drawing. Whether it’s a flowchart, mind map, or simple doodle, it might just help you break down the walls between you and that elusive solution.

Using logical reasoning and critical thinking

You’re already using such great strategies, but let’s not forget about the power of good, old-fashioned logic and critical thinking. They are the backbone of effective problem solving. Here’s what they can do for you:

Breaks down complex problems: Logical reasoning enables you to dissect big issues into smaller, more manageable parts.

Facilitates decision making: By evaluating information critically, you can make more informed decisions.

Reduces bias: Critical thinking encourages you to question assumptions and explore multiple viewpoints, leading to less biased and more objective conclusions.

When your next challenge arises, remember to ask the tough questions and follow the trail of logic. Your keen sense of reason might just be the key to unlocking the solution!

Remember, every problem is different, and there’s no one-size-fits-all technique. Try blending these strategies with those you’re already rocking, and you’ll become an unstoppable problem-solving force! Keep it up!

Problem Solving in Different Mathematical Concepts

Problem solving in algebra.

Have you ever found yourself befuddled by x’s and y’s swarming in your textbook? Fear not, as algebra often becomes friendlier when you employ some problem-solving finesse. Here’s how you can tackle algebraic puzzles like a pro:

• Find common patterns : Algebra is all about recognizing patterns. Stay on the lookout for familiar equations or formulas that can simplify your work.
• Work backwards : Stuck on where to start? Try working backwards from the answer. This reverse-engineering approach can reveal insights into the steps needed.
• Use substitution : When equations get messy, substituting variables with numbers or simpler expressions can make life a whole lot easier.
• Implement these tips during your next algebra session, and watch those variables bow down to your newfound strategic prowess!

Problem Solving in Geometry

Shifting gears to geometry, where shapes and angles reign supreme, the right strategies can unveil the mysteries behind those theorems and postulates:

• Draw it out : Never underestimate the power of a good sketch. Often, drawing the problem can reveal solutions that aren’t immediately obvious in text form.
• Break it down : Complex geometric figures can be intimidating. Break them into smaller parts, and focus on solving each section at a time.
• Look for congruencies and similarities : Pattern recognition isn’t only useful in algebra. In geometry, identifying congruent angles and similar triangles can save you loads of time. Next time you’re faced with tangents and secants, remember that a ruler and compass are your trusty sidekicks on this geometric adventure.

Embrace these approaches when you next encounter mathematical head-scratchers. Each concept might require a unique touch, but your creative thinking and analytical skills will shine through no matter the equation or theorem!

Tips for Effective Problem Solving

Breaking down complex problems into smaller steps.

You’ve got this! When faced with a complicated problem, don’t let it overwhelm you. Take a deep breath and start by breaking it down into smaller, more manageable pieces. This approach makes the problem less intimidating and allows you to focus on one piece at a time.

Approach each step one at a time : Patience is key here. Concentrate on solving each individual part of the problem before trying to tackle it as a whole. This step-by-step process can prevent mistakes and lead you to the correct solution more efficiently.

Celebrate small victories : Every time you complete a step, give yourself a pat on the back. These mini wins help maintain your motivation and keep your spirits high.

Utilizing past problem solving experiences

Think back to similar challenges. Remember that algebra equation you conquered last week? Draw on the strategies that worked for you then. Your past successes are a treasure trove of wisdom.

Learn from previous mistakes : It’s okay if you didn’t get it right the first time. What’s important is to reflect on what went wrong and use that insight to inform your current approach.

Collaboration is your friend : If you’re truly stumped, don’t hesitate to ask for help. Maybe your peers or teachers have faced a similar issue before. Their experience could provide you the breakthrough you need.

Remember, every problem you face is an opportunity to sharpen your problem-solving skills. You’re capable of tackling each one, especially when you apply tried-and-true strategies tailored to the task at hand. Keep going, keep learning, and most importantly, keep believing in yourself!

Start Small: Don’t let a big problem scare you. Begin by splitting it up into bite-sized parts you can handle. Smaller issues are less daunting and easier to solve.

One Step at a Time: Tackle one piece of the puzzle before moving on to the next. This methodical strategy helps you stay focused and makes the overall problem solvable.

Celebrate Progress: Each completed step is a victory. Recognize these achievements to stay motivated on your problem-solving journey.

Recall Previous Successes: Think about how you’ve solved problems before. Use those strategies again—they’re part of your personal toolkit now.

Learn From Mistakes: It’s fine if things didn’t work out last time. Reflect on what happened and apply those lessons to your present challenge.

Seek Help When Needed: If you’re stuck, reach out. Others around you might have insights from their own experiences that can help you break through.

Remember that with every problem you come across, you get a chance to improve your problem-solving skills . You’ve got what it takes to overcome these challenges, especially when you apply proven strategies that suit the situation. Keep pushing forward, keep learning, and most importantly, keep believing in yourself!

• Grade 1 Lessons
• Grade 2 Lessons
• Grade 3 Lessons
• Grade 4 Lessons
• Grade 5 Lessons
• Grade 6 Lessons
• Grade 7 Lessons
• Grade 8 Lessons
• Kindergarten
• Math Activities
• Math Lessons Online
• Multiplication
• Subtraction
• #basic mathematic
• #Basic Mathematical Operation
• #best math online math tutor
• #Best Math OnlineTutor
• #dividing fractions
• #effective teaching
• #grade 8 math lessons
• #linear equation
• #Math Online Blog
• #mathematical rule
• #mutiplying fractions
• #odd and even numbers
• #Online Math Tutor
• #online teaching
• #order of math operations
• #pemdas rule
• #Point-Slope Form
• #Precalculus
• #Slope-Intercept Form
• #Tutoring Kids

Thank you for signing up!

GET IN TOUCH WITH US