definition of kinetic energy essay

• Science Notes Posts
• Contact Science Notes
• Todd Helmenstine Biography
• Anne Helmenstine Biography
• Free Printable Periodic Tables (PDF and PNG)
• Periodic Table Wallpapers
• Interactive Periodic Table
• Periodic Table Posters
• Science Experiments for Kids
• How to Grow Crystals
• Chemistry Projects
• Fire and Flames Projects
• Holiday Science
• Chemistry Problems With Answers
• Physics Problems
• Unit Conversion Example Problems
• Chemistry Worksheets
• Biology Worksheets
• Periodic Table Worksheets
• Physical Science Worksheets
• Science Lab Worksheets
• My Amazon Books

What Is Kinetic Energy? Kinetic Energy Examples

Potential and kinetic energy are the two major types of energy . Here is a look at kinetic energy, including its definition, examples, units, formula, and how to calculate it.

Kinetic Energy Definition

In physics, kinetic energy is the energy an object has due to its motion. It is defined as the work required to accelerate a body of a given mass from rest to a certain velocity. Once the mass reaches the velocity, its kinetic energy remains unchanged unless its speed changes. However, velocity and thus kinetic energy depend on the frame of reference. In other words, an object’s kinetic energy is not invariant.

Kinetic Energy Units

The SI unit of kinetic energy is the joule (J), which is a kg⋅m 2 ⋅s −2 . The English unit of kinetic energy is the foot-pound (ft⋅lb). Kinetic energy is a scalar quantity . It has magnitude, but no direction.

Kinetic Energy Examples

Anything you can think of that has mass (or apparent mass) and motion is an example of kinetic energy. Kinetic energy examples include:

• A flying aircraft, bird, or superhero
• Walking, jogging, bicycling, swimming, dancing, or running
• Falling down or dropping an object
• Throwing a ball
• Driving a car
• Playing with a yo-yo
• Launching a rocket
• A windmill spinning
• Clouds moving across the sky
• An avalanche
• A waterfall or flowing stream
• Electricity flowing through a wire
• Orbiting satellites
• A meteor falling to Earth
• Sound moving from a speaker to your ears
• Electrons orbiting the atomic nucleus
• Light traveling from the Sun to the Earth (photons have momentum, so they have apparent mass)

Kinetic Energy Formula

The formula for kinetic energy (KE) relates energy to mass (m) and velocity (v).

KE = 1/2 mv 2

Because mass is always a positive value and the square of any value is a positive number, kinetic energy is always positive. Also, this means the maximum kinetic energy occurs when velocity is greatest, regardless of the direction of motion.

From the kinetic energy equation, you can see an object’s velocity matters more than its mass. So, even a small object has a lot of kinetic energy if it’s moving quickly.

The kinetic energy formula works in classical physics, but it starts to deviate from true energy when the velocity approaches the speed of light ( c ).

How to Calculate Kinetic Energy

The key to solving kinetic energy problems is to remember that 1 joule equals 1 kg⋅m 2 ⋅s −2 . Speed is the magnitude of velocity, so you can use it in the kinetic energy equation. Otherwise, watch your units in fractions. For example, (1)/(400 m 2 /s 2 ) is the same as (1/400) s 2 /m 2 .

Calculate the kinetic energy of a 68 kg person moving with a speed of 1.4 m/s (in other words, the kinetic energy of a typical person walking).

Plugging in the numbers:

KE = 1/2(68 kg)(1.4 m/s) 2 KE = 66.64 kg⋅m 2 ⋅s −2 KE = 66.64 J

Calculate the mass of an object moving at 20 m/s with a kinetic energy of 1000 J.

Rearrange the kinetic energy equation to solve for mass:

m = 2KE/v 2 m = (2)(1000 kg⋅m 2 ⋅s −2 )/(20 m/s) 2 m= (2000 kg⋅m 2 ⋅s −2 )/(400 m 2 /s 2 ) m = 5 kg

Difference Between Kinetic and Potential Energy

Kinetic energy can transform into potential energy , and vice versa. Kinetic energy is the energy associated with a body’s motion, while potential energy is the energy due to an object’s position. All the other types of energy (e.g., electrical energy , chemical energy , thermal energy, nuclear energy) have kinetic energy, potential energy, or a combination of the two. The sum of the kinetic and potential energy of a system (its total energy) is a constant because of Conservation of Energy . In quantum mechanics, the sum of kinetic and potential energy is called the Hamiltonian.

A frictionless roller coaster is a good example of the interplay between kinetic and potential energy . At the top of the track, the roller coaster has maximum potential energy, but minimum kinetic energy (zero). As the cart goes down the track, its velocity increases. At the bottom of the track, the potential energy is at its minimum (zero), while the kinetic energy is at its maximum.

• Goel, V. K. (2007). Fundamentals Of Physics . Tata McGraw-Hill Education. ISBN 978-0-07-062060-5.
• Serway, Raymond A.; Jewett, John W. (2004). Physics for Scientists and Engineers (6th ed.). Brooks/Cole. ISBN 0-534-40842-7.
• Tipler, Paul; Llewellyn, Ralph (2002). Modern Physics (4th ed.). W. H. Freeman. ISBN 0-7167-4345-0.

Most Popular

Evernote review, kinetic energy essay sample, example.

The technical term for the energy associated with motion is kinetic energy, from the Greek word for motion (the root is the same as the root of the word “cinema” for a motion picture, and in French the term for kinetic energy is “énergie cinétique”). To find how much kinetic energy is possessed by a given moving object, we must convert all its kinetic energy into heat energy, which we have chosen as the standard reference type of energy. We could do this, for example, by firing projectiles into a tank of water and measuring the increase in temperature of the water as a function of the projectile’s mass and velocity. Consider the following data from a series of three such experiments:

m (kg) v (m/s) energy (J) 1.00 1.00 0.50 1.00 2.00 2.00 2.00 1.00 1.00

Comparing the first experiment with the second, we see that doubling the object’s velocity does not just double its energy, it quadruples it. If we compare the first and third lines, however, we find that doubling the mass only doubles the energy. This suggests that kinetic energy is proportional to mass and to the square of velocity, KE∝mv2KE∝mv2, and further experiments of this type would indeed establish such a general rule. The proportionality factor equals 0.5 because of the design of the metric system, so the kinetic energy of a moving object is given by

The metric system is based on the meter, kilogram, and second, with other units being derived from those. Comparing the units on the left and right sides of the equation shows that the joule can be reexpressed in terms of the basic units as kg⋅m2/s2kg⋅m2/s2.

Students are often mystified by the occurrence of the factor of 1/2, but it is less obscure than it looks. The metric system was designed so that some of the equations relating to energy would come out looking simple, at the expense of some others, which had to have inconvenient conversion factors in front. If we were using the old British Engineering System of units, then we would have the British Thermal Unit (BTU) as our unit of energy. In that system, the equation you would learn for kinetic energy would have an inconvenient proportionality constant, KE=(1.29×10−3)mv2KE=(1.29×10−3)mv2, with KEKE measured in units of BTUs, vv measured in feet per second, and so on. At the expense of this inconvenient equation for kinetic energy, the designers of the British Engineering System got a simple rule for calculating the energy required to heat water: one BTU per degree Fahrenheit per pound. The inventor of kinetic energy, Thomas Young, actually defined it as KE=mv2KE=mv2, which meant that all his other equations had to be different from ours by a factor of two. All these systems of units work just fine as long as they are not combined with one another in an inconsistent way.

Example: Energy released by a comet impact

– Comet Shoemaker-Levy, which struck the planet Jupiter in 1994, had a mass of roughly 4×10134×1013 kg, and was moving at a speed of 60 km/s. Compare the kinetic energy released in the impact to the total energy in the world’s nuclear arsenals, which is 2×10192×1019 J. Assume for the sake of simplicity that Jupiter was at rest.

– Since we assume Jupiter was at rest, we can imagine that the comet stopped completely on impact, and 100% of its kinetic energy was converted to heat and sound. We first convert the speed to mks units, v=6×104v=6×104 m/s, and then plug in to the equation to find that the comet’s kinetic energy was roughly 7×10227×1022 J, or about 3000 times the energy in the world’s nuclear arsenals.

Is there any way to derive the equation KE=(1/2)mv2KE=(1/2)mv2 mathematically from first principles? No, it is purely empirical. The factor of 1/2 in front is definitely not derivable, since it is different in different systems of units. The proportionality to v2v2 is not even quite correct; experiments have shown deviations from the v2v2 rule at high speeds, an effect that is related to Einstein’s theory of relativity. Only the proportionality to mm is inevitable. The whole energy concept is based on the idea that we add up energy contributions from all the objects within a system. Based on this philosophy, it is logically necessary that a 2-kg object moving at 1 m/s have the same kinetic energy as two 1-kg objects moving side-by-side at the same speed.

Energy and relative motion

Although I mentioned Einstein’s theory of relativity above, it is more relevant right now to consider how the conservation of energy relates to the simpler Galilean idea that motion is relative. Galileo’s Aristotelian enemies (and it is no exaggeration to call them enemies!) would probably have objected to conservation of energy. After all, the Galilean idea that an object in motion will continue in motion indefinitely in the absence of a force is not so different from the idea that an object’s kinetic energy stays the same unless there is a mechanism like frictional heating for converting that energy into some other form.

More subtly, however, it is not immediately obvious that what we have learned so far about energy is strictly mathematically consistent with the principle that motion is relative. Suppose we verify that a certain process, say the collision of two pool balls, conserves energy as measured in a certain frame of reference: the sum of the balls’ kinetic energies before the collision is equal to their sum after the collision (in reality, we would need to add in other forms of energy, like heat and sound, that are liberated by the collision, but let us keep it simple). But what if we were to measure everything in a frame of reference that was in a different state of motion? A particular pool ball might have less kinetic energy in this new frame; for example, if the new frame of reference was moving right along with it, its kinetic energy in that frame would be zero. On the other hand, some other balls might have a greater kinetic energy in the new frame. It is not immediately obvious that the total energy before the collision will still equal the total energy after the collision. After all, the equation for kinetic energy is fairly complicated, since it involves the square of the velocity, so it would be surprising if everything still worked out in the new frame of reference. It does still work out.

Follow us on Reddit for more insights and updates.

Comments (0)

Welcome to A*Help comments!

We’re all about debate and discussion at A*Help.

We value the diverse opinions of users, so you may find points of view that you don’t agree with. And that’s cool. However, there are certain things we’re not OK with: attempts to manipulate our data in any way, for example, or the posting of discriminative, offensive, hateful, or disparaging material.

Cancel reply

Your email address will not be published. Required fields are marked *

Save my name, email, and website in this browser for the next time I comment.

More from Definition Essay Examples and Samples

Oct 23 2023

What Is Respect? Definition Essay Example

Oct 18 2023

What Is Family? Definition Essay Samples

Oct 17 2023

What Is Integrity? Essay Definition Example

Related writing guides, writing a definition essay.

Remember Me

What is your profession ? Student Teacher Writer Other

Forgotten Password?

Username or Email

• Why Does Water Expand When It Freezes
• Gold Foil Experiment
• Faraday Cage
• Oil Drop Experiment
• Magnetic Monopole
• Why Do Fireflies Light Up
• Types of Blood Cells With Their Structure, and Functions
• The Main Parts of a Plant With Their Functions
• Parts of a Flower With Their Structure and Functions
• Parts of a Leaf With Their Structure and Functions
• Why Does Ice Float on Water
• Why Does Oil Float on Water
• How Do Clouds Form
• What Causes Lightning
• How are Diamonds Made
• Types of Meteorites
• Types of Volcanoes
• Types of Rocks

Kinetic Energy

What is kinetic energy.

The energy acquired by an object due to its motion is known as kinetic energy. The motion can be translational, rotational, vibrational, or a combination of all three.

According to Newton’s First Law , an object at rest will stay at rest unless acted by force. When force is applied, it does work on the object. Work means the transfer of energy from one form to another. In this case, the form into which energy transforms is kinetic energy. The object attains kinetic energy and moves with a specific velocity .

Kinetic Energy Equation

How to find kinetic energy.

Kinetic energy is a scalar quantity, not a vector. It takes positive values that depend on two factors – velocity and mass. It cannot be negative since mass cannot be negative. The formula for kinetic energy is given by,

K.E. = ½ mv 2

K.E. : kinetic energy

v : velocity

SI Unit: Joule or J ( 1 J = 1 kg m 2 /s 2 )

Cgs Unit : Erg ( 10 7 erg = 1 J )

Dimensions : [ML 2 T -2 ]

For the above equation, it is clear that increasing velocity increases kinetic energy. Similarly, an object with a higher mass will have higher kinetic energy.

Change in Kinetic Energy

An object can start from rest and acquire velocity. An object can also change its velocity during its motion. Suppose the object moves with a velocity v i , the initial velocity. A force alters its motion such that its velocity changes to v f , which is the final velocity. Then, the change in kinetic energy is,

Δ K.E. = ½ mv f 2 – ½ mv i 2

Now, work is being done on the object to change its motion. The change of kinetic energy is equal to the work done W . Therefore,

This equation is known as the work-energy theorem.

Rotational Kinetic Energy

An object rotating about its axis also has kinetic energy. Its kinetic energy depends on angular velocity and moment of inertia . For rotational motion , the expression for kinetic energy is different from linear motion. The formula is,

K.E. = ½ I ω 2

I : moment of inertia

ω : angular velocity

An example of rotational kinetic energy is a merry-go-round.

Suppose an object executes both translational and rotational motion. In that case, the total kinetic energy is the sum of kinetic energy due to each motion.

K.E. Total = ½ mv 2 + ½ Iω 2

Vibrational Kinetic Energy

A vibrating object has kinetic energy. For example, a spring oscillates with kinetic energy given by,

K.E. = ½ kx 2

k : spring constant

x : displacement

Kinetic Energy Examples

Any object that has mass is capable of moving has kinetic energy. Here are a few examples.

• A bird flying in the air
• A ship sailing in the sea
• A car driving on the road
• A rocket launching into space
• Water flowing down a stream
• Planets orbiting around the sun with a gravitational force
• A windmill with its blades rotating
• A bullet fired from a gun
• An oscillating pendulum
• An archer releasing an arrow from a bow
• A roller coaster in descent

The following image shows a few examples of kinetic energy.

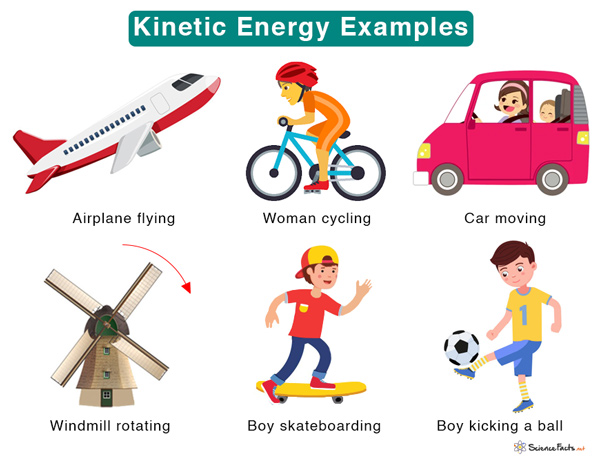
Types of Kinetic Energy

There are five different forms of kinetic energy.

1. Mechanical Energy : It is the energy associated with the mechanical movement of objects.

• A bowling ball rolling down an alley
• A soccer ball moving through the air

2. Electrical Energy : Also known as electricity, it is caused by electron flow along a conductor.

• A table lamp turned on
• Electrolysis

3. Sound Energy : It is caused by the motion of vibrating air particles. When the vibrations reach the ears, the brain perceives them as sound.

• Human voice
• Sound of drums

4. Radiant Energy : It is caused by oscillating electric and magnetic fields, resulting in electromagnetic waves . Particles or waves carry the energy.

• Light emitted by incandescent light bulbs
• Radiation emitted by X-rays

5. Thermal Energy : Also known as heat energy, it is caused by atoms and molecules moving and colliding. The faster the atoms move, the hotter the object will be.

• Boiling water in a pan
• Baking food in an oven

The following points summarize all that we have learned about kinetic energy.

• Associated with a moving object
• Increases or decreases with the change in velocity
• Greater for heavier mass
• Can transform into other types of energy
• Measured in the unit of Joules

Solved Problems

P.1. A ball weighs around 2 kg and travels at 15 meters per second. What is its kinetic energy?

Soln.: Given,

K.E. = ½ (2 kg) (15 m/s) 2

Or, K.E. = 225 J

P.2. Calculate the kinetic energy of a 78 kg person walking with a speed of 1.2 m/s?

v = 1.2 m/s

Or, K.E. = ½ (78 kg) (1.2 m/s) 2

Or, K.E. = 56.2 J

P.3. Calculate the mass of an object moving at 25 m/s with a kinetic energy of 1500 J.

Soln. : Given,

K.E. = 1500 J

The kinetic energy is,

Or, m = 2 K.E./v 2

Or, m = 2 x 1500 kg m 2 s -2 /(25 ms – ) 2

Or, m = 4.8 kg

P.4. Work done by a force on a moving object is 150 J. The object was traveling at a speed of 2.5 m/s. Find the new speed of the object if the mass of the object is 1.5 kg.

v i = 2.5 m/s

Or, W = ½ m (v f 2 – v i 2 )

Or, v f 2 = 2W/m + v i 2

Or, v f 2 = 2 x 150 kg ms -1 /1.5 kg + (2.5 ms -1 ) 2 = 206 m 2 /s s

Or, v f = √206 m 2 /s s = 14.3 m/s

Ans. Kinetic energy is not a stored energy. Rather, it is a release energy.

Ans. An example of an object that can absorb kinetic energy is aluminum.

Ans. A source of kinetic energy is wind.

• What is Kinetic Energy? – Khanacademy.org
• Kinetic Energy – Hyperphysics.phy-astr.gsu.edu
• Kinetic – Ducksters.com
• What is Kinetic Energy? – Livescience.com
• Kinetic Energy – Physics.info
• What is Kinetic Energy? Kinetic Energy Examples – Sciencenotes.org

Article was last reviewed on Monday, February 7, 2022

Related articles

Leave a Reply Cancel reply

Your email address will not be published. Required fields are marked *

Save my name, email, and website in this browser for the next time I comment.

Popular Articles

Join our Newsletter

Fill your E-mail Address

Related Worksheets

• Privacy Policy

© 2024 ( Science Facts ). All rights reserved. Reproduction in whole or in part without permission is prohibited.

• Science, Tech, Math ›
• Chemistry ›
• Chemical Laws ›

The Definition of Kinetic Energy

• Chemical Laws
• Periodic Table
• Projects & Experiments
• Scientific Method
• Biochemistry
• Physical Chemistry
• Medical Chemistry
• Chemistry In Everyday Life
• Famous Chemists
• Activities for Kids
• Abbreviations & Acronyms
• Weather & Climate
• Ph.D., Biomedical Sciences, University of Tennessee at Knoxville
• B.A., Physics and Mathematics, Hastings College

Kinetic energy is the energy an object possesses due to its motion. An object of mass m moving at velocity v has a kinetic energy equal to ½mv 2 .

An example of kinetic energy is a swinging pendulum.

• Joule Definition (Unit in Science)
• Evaporation Definition in Chemistry
• Gases Study Guide
• Chemical Kinetics Definition in Chemistry
• Internal Energy Definition
• Enthalpy Change Definition in Science
• The Law of Conservation of Energy Defined
• Potential Energy Definition and Formula
• What Is the Ideal Gas Law?
• Ideal Gas Definition
• Heat Capacity Definition
• Intermolecular Force Definition in Chemistry
• Understanding Electrical, Thermal, and Sound Conductors
• Reaction Rate Definition in Chemistry
• What Is the Difference Between Diffusion and Effusion?
• What Is Absolute Zero in Science?

• Physics class-9 Notes
• Physics Formulas
• Physics Symbol
• Application of Physics
• Class 8 Science
• Class 9 Science
• Class 10 Science
• Class 11 Science
• Class 12 Science
• Class 8 Study Material
• Class 9 Study Material
• Class 10 Study Material
• Class 11 Study Material
• Class 12 Study Material

Kinetic Energy

Kinetic Energy is the energy associated with an object moving with a velocity. For an object of mass m and velocity, its kinetic energy is half of the product of the mass of the object with the square of its velocity. In our daily life, we observe kinetic energy while walking, cycling, throwing a ball, and others.

Learn Kinetic Energy and others in detail.

Table of Content

What is Kinetic Energy?

Unit of kinetic energy, kinetic energy examples, is kinetic energy a vector or a scalar quantity, kinetic energy transformation, kinetic energy formula, derivation for kinetic energy equation.

Kinetic energy of an object is defined as the energy that is generated due to the motion of the object. The kinetic energy by an object arises when it is allowed to accelerate, it requires the application of some forces on it that leads to the work done. Therefore, after the work is done, the energy is transferred to the objects that lead to the motion of the object at a constant velocity. The energy that is transferred is called kinetic energy which totally depends on the speed and mass of the object in motion.

An object that is moving in a certain direction can do work. An object that is moving faster can do more work than an identical object that is moving relatively slowly.

Examples: Some of the common examples of Kinetic Energy are, a moving bullet, blowing wind, a speeding stone, a rotating wheel, arrow released from the bow that has kinetic energy.

Objects in motion possess energy. This energy is simply known to be kinetic energy. A falling coconut, a flying aircraft, a speeding car, flowing water, a rolling stone, blowing wind, a running athlete, etc. i.e. any moving object possesses kinetic energy.

In short, kinetic energy is the energy possessed by an object due to its motion. The kinetic energy of an object increases when the speed of the object increase.

Check: Define Kinetic Energy, give two Examples of Kinetic Energy.

Kinetic energy is measured in various units which are ,

SI Unit: SI unit of kinetic energy is Joule (J) or kg.m 2 .s -2 . CGS Unit: In CGS system, the kinetic energy is defined in erg.

The dimension Formula for kinetic energy is [M 1 L 2 T -2 ]

Various examples of Kinetic Energy are,

• A truck travelling down the road has more kinetic energy than a car travelling at the same speed because the truck’s mass is much more than the car’s.
• Kinetic Energy of an asteroid falling towards the earth is very large as it has a large mass and very high velocity.
• A bullet has very high kinetic energy as its speed is very high.

Mass (m) is a scalar quantity and velocity (v) is a vector quantity. The formula for kinetic energy is,

K = 1/2 m×v 2

From the above formula, it is observed that velocity is squared, and it is known that the square of any vector quantity is a scalar quantity. 

Hence, kinetic energy is a scalar quantity.

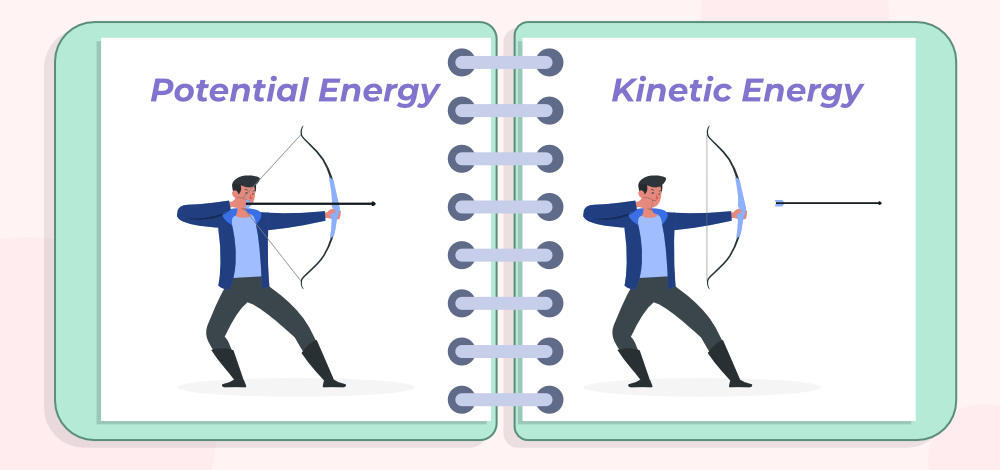
• Kinetic energy transformation is possible between objects and also among different forms of energy. Dropping the ball is a very good example of kinetic energy transformation.
• When the ball is resting in hand, the energy contained in the ball is the potential energy due to its height.
• When it is dropped, the potential energy starts converting into kinetic energy due to its motion. When the ball is about to reach the ground, the entire potential energy is converted into kinetic energy.

Check : Rotational Kinetic Energy

As the kinetic energy of an object depends on its mass and speed therefore mathematically, the kinetic energy is defined as,

K = 1/2 m×v 2 where, m is the mass of the object, v is the speed or velocity of the object

This, expression obtained is called the Kinetic Energy Equation .

Check : Potential Energy Formula

Consider an object having mass m, initial velocity, u, and final velocity, v.

Suppose when a constant force, F is applied to it, it displaces to a distance s.

Now, work is done by the object that is responsible to change in its velocity, the work done is:

W = F × s ……(1)

Let its velocity change from u to v and a is the acceleration produced.

Now, using the equation of motion that relates u, v, s, and an as,

v 2 – u 2 = 2as

Solving the above expression for s as

[Tex]s=\dfrac{v^2-u^2}{2a}[/Tex]

But it is known that the net force acting on an object is defined as:

F = ma Now, Substitute ma for F and  [Tex]\dfrac{v^2-u^2}{2a} [/Tex]  for s in the equation (1) and solve to calculate W. [Tex]\begin{aligned}W&=(ma)\left(\frac{v^2-u^2}{2a}\right)\\&=\dfrac{m(v^2-u^2)}{2}\end{aligned}[/Tex] Suppose the object is starting from its initial position, i.e. u = 0, then: [Tex]\begin{aligned}W&=\dfrac{m(v^2-(0)^2)}{2}\\&=\dfrac{1}{2}mv^2\end{aligned}[/Tex] It is clear that the work done is always equal to the change in the kinetic energy of an object. So, the kinetic energy possessed by an object of mass, m and moving with a uniform velocity, v is [Tex]\begin{aligned}\text{K.E.}=\dfrac{1}{2}mv^2\end{aligned}[/Tex]

Types of Kinetic Energy

There are five types of kinetic energy that are,

Radiant Energy

Thermal energy, sound energy, electrical energy, mechanical energy.

Radiant energy is a form of kinetic energy, which is the physical energy of matter resulting from electromagnetic radiation. It is radiated from matter into the surrounding environment.

For example, Ultraviolet light, Gamma rays, and X-rays all have electromagnetic radiant energy.

Thermal energy can be also known as heat energy. It is generated by the kinetic energy of the motion of atoms when they collide with each other.

For Example, Hot Springs, Heated Swimming pools have thermal energy.

Sound energy is the energy, produced by the vibration of an object. It travels through the medium but cannot travel in a vacuum or space, as there are no particles to act as a medium.

For Example, Tuning Forks, Beating Drums have sound energy.

Electrical energy can be obtained from the free electrons that are of positive and negative charge. 

For Example, Batteries have electrical energy.

The sum of kinetic energy and potential energy is called mechanical energy. It can neither be created nor destroyed but it can be converted from one form to other.

For Example, Satellites orbiting around the Earth, Moving Cars all have mechanical energy.

Difference Between Kinetic Energy and Potential Energy

The difference between Kinetic Energy and Potential Energy is discussed below,

• Kinetic energy is the energy that an object possesses due to its motion. It is defined as half of the product of the object’s mass and its velocity squared. In other words, the more massive an object is and the faster it moves, the greater its kinetic energy.

The formula for kinetic energy is: Kinetic energy = 1/2 x mass x velocity^2

• where “ mass” is the mass of the object and “velocity” is its speed in meters per second.
• The unit of kinetic energy is joules (J) in the International System of Units (SI). However, other units such as calories, ergs, or electronvolts can also be used to express kinetic energy.
• Kinetic energy is a scalar quantity, which means it has magnitude but no direction. It is also a conserved quantity, which means that it can be transferred between objects or transformed into other forms of energy, but the total amount of kinetic energy in a closed system remains constant.
• Kinetic energy is important in many areas of physics and engineering, including mechanics, thermodynamics, and electromagnetism.
• It is used to calculate the work done by a force on an object, the momentum of an object, and the temperature of a gas. In everyday life, kinetic energy is involved in a wide range of phenomena, such as the movement of vehicles, the flight of birds, and the vibration of molecules in a gas.

Difference between Kinetic Energy and Potential Energy

Solved Examples on Kinetic Energy

Example 1: A vehicle having a mass of 150 kg, is moving with a uniform velocity of 4 m/s. What is the amount of kinetic energy possessed by the vehicle?

Given, Mass of the vehicle, m = 150 kg, Velocity of the vehicle, v = 4 m/s Kinetic of the vehicle is,  [Tex]\begin{aligned}\text{K.E.}&=\dfrac{1}{2}mv^2\\&=\dfrac{1}{2}\times150\text{ kg}\times(4\text{ m/s})^2\\&=1200\text{ J}\end{aligned}[/Tex] Hence, the kinetic energy of the vehicle is equal to 1200 J.

Example 2: A ball having a mass of 2 kg is thrown up with a speed of 10 m/s. What is the kinetic energy stored in the ball at the time of throwing?

Given, Mass of the ball, m = 2 kg, Velocity of the ball, v = 10 m/s. Kinetic of the ball is,  [Tex]\begin{aligned}\text{K.E.}&=\dfrac{1}{2}mv^2\\&=\dfrac{1}{2}\times2\text{ kg}\times(10\text{ m/s})^2\\&=100\text{ J}\end{aligned}[/Tex] Hence, the kinetic energy of the ball is equal to 100 J.

Example 3: An asteroid is coming towards the earth. Its velocity is 1000 km/s. Its estimated kinetic energy is almost 4 × 10 15 J. Find out the mass of the asteroid.

Given, Kinetic energy of the asteroid, K.E. = 4 × 10 15 J, Velocity of the asteroid, v = 1000 Km/s = 10 6 m/s Kinetic of the asteroid is given as,  [Tex]\begin{aligned}\text{K.E.}&=\dfrac{1}{2}mv^2\\4\times10^{15}\text{ J}&=\dfrac{1}{2}\times m\times(10^6\text{ m/s})^2\\&m=8000\text{ kg}\end{aligned}[/Tex] Hence, the mass of the asteroid is equal to 8000 kg.

Related Article

Practice Problems on Kinetic Energy What is Energy? Real Life Applications of Kinetic Energy

Conclusion of Kinetic Energy

Kinetic energy is essential to both the operation of the natural world and our daily life. It is the energy that moving objects possess and is necessary for a number of operations and occurrences, including the flow of rivers, the movement of machinery and cars, and bird flight. Knowing about kinetic energy helps us to understand basic physics ideas like momentum and the conservation of energy, as well as to use it for useful purposes like power generation via wind turbines or hydroelectric dams. The effective use of kinetic energy will remain essential for sustainable development and the evolution of human civilization as long as we keep inventing and exploring new technologies.

Kinetic Energy FAQs

Kinetic Energy is the energy posses by the object due to virtue of its motion.

What is the Kinetic Energy of an Object?

The kinetic energy of an object is given by the formula, K.E. = 1/2 mv 2

How does the Average Kinetic Energy of molecules depend on Absolute Temperature?

The average kinetic energy of any molecule depends directly on absolute temperature as the increase in absolute temperature increases the kinetic energy of the molecules.

Can the kinetic energy be negative?

Kinetic Energy of an object can never be negative as it is given by the formula K.E. = 1/2 mv 2 here, mass can never be negative and the square of any number is always positive.

When is the Kinetic Energy Maximum?

Kinetic energy of an object is maximum when the potential energy of the object is minimum, and the velocity of the object is maximum.

What happens to the kinetic energy of an object when the speed is doubled?

When the speed of an object is doubled its kinetic energy is increased four times as kinetic energy is directly proportional to the square of the speed.

What is the difference between Kinetic Energy and Potential Energy?

Kinetic energy is the energy possessed by any particle due to its motion (speed) whereas potential energy is the energy of an object due to its position.

Similar Reads

• CBSE Class 11 Physics Notes CBSE Class 11 Physics Notes 2023-24 is a comprehensive guide for CBSE Class 11 students. The class 11 syllabus is designed to provide students with a strong foundation in the basic principles of physics, including Measurement, Vectors, Kinematics, Dynamics, Rotational Motion, Laws of Motion, and Gra 12 min read

Chapter 1 - UNITS AND MEASUREMENT

• Measurement Measurement is a technique that is required to measure and quantify various parameters of an object. Measurement is the essential metric to express any quantity of objects, things, and events that help us to compare that object with other similar objects. In our daily lives, we use measurement in va 12 min read
• System of Units Measurement forms the fundamental principle to various other branches of science, that is, construction and engineering services. Measurement is defined as the action of associating numerical with their possible physical quantities and phenomena. Measurements find a role in everyday activities to a 9 min read
• Significant Figures In Order to find the value of different sizes and compare them, measurement is used. Measuring things is not only a concept but also practically used in everyday life, for example, a milkman measures milk before selling it in order to make sure the correct amount is served, A tailor always measures 6 min read
• Units and Dimensions Units and Dimensions is a fundamental and essential topic in Physics. For the measurement of a physical quantity, Unit plays a vital role. Unit provides a complete idea about the measurement of a physical quantity. Dimension is a measure of the size or extent of a particular quantity. In this articl 8 min read
• Dimensional Formula Dimensional Formulas play an important role in converting units from one system to another and find numerous practical applications in real-life situations. Dimensional Formulas are a fundamental component of the field of units and measurements. In mathematics, Dimension refers to the measurement of 9 min read
• Dimensional Analysis Most of the physical things are measurable in this world. The system developed by humans to measure these things is called the measuring system. Every measurement has two parts, a number (n) and a unit(u). The unit describes the number, what this number is and what it signifies. For example, 46 cm, 6 min read

Chapter 2 - MOTION IN A STRAIGHT LINE

• What is Motion? Motion is defined as the change in the position of an object with respect to time i.e. when an object changes its position according to time it is said to be in the state of motion. Everything in the universe is in a state of continuous motion, for example, the moon revolves around the planets, the 12 min read
• Instantaneous Velocity Formula The speed of a moving item at a given point in time while retaining a specific direction is known as instantaneous velocity. With the passage of time, the velocity of an object changes. On the other hand, velocity is defined as the ratio of change in position to change in time when the difference in 4 min read
• Instantaneous Speed Formula Velocity is defined as the rate of change of its position with respect to its frame of reference. It is a vector quantity as it has magnitude and direction. The SI unit of velocity is meter per second or m/s.Whereas speed measures the distance traveled by an object over the change in time. It has ma 5 min read
• Acceleration Acceleration is defined as the rate of change in velocity. This implies that if an object’s velocity is increasing or decreasing, then the object is accelerating. Acceleration has both magnitude and direction, therefore it is a Vector quantity. According to Newton's Second Law of Motion, acceleratio 9 min read
• Uniform Acceleration Uniformly Accelerated Motion or Uniform Acceleration in Physics is a motion in which the object is accelerated at constant acceleration. We have to keep in mind that uniform accelerated motion does not mean uniform velocity i.e. in uniform accelerated the velocity of the object increases linearly wi 8 min read
• Relative Velocity Formula Let us suppose we are travelling on a Bus, let's say, another bus overtakes us. We will not feel the actual speed of the overtaking bus, as felt by a person who looks it, standing by the side of the road. If both the buses are moving at the same speed in the same direction, a person in one bus obser 10 min read

Chapter 3 - MOTION IN A Plane

• Scalar and Vector Scalar and Vector Quantities are used to describe the motion of an object. Scalar Quantities are defined as physical quantities that have magnitude or size only. For example, distance, speed, mass, density, etc. However, vector quantities are those physical quantities that have both magnitude and di 8 min read
• Product of Vectors Vector operations are used almost everywhere in the field of physics. Many times these operations include addition, subtraction, and multiplication. Addition and subtraction can be performed using the triangle law of vector addition. In the case of products, vector multiplication can be done in two 6 min read
• Vector Operations Vector Operations are operations that are performed on vector quantities. Vector quantities are the quantities that have both magnitude and direction. So performing mathematical operations on them directly is not possible. So we have special operations that work only with vector quantities and hence 9 min read
• Resolution of Vectors Vector Resolution is splitting a vector into its components along different coordinate axes. When a vector is expressed in terms of its components, it becomes easier to analyze its effects in different directions. This process is particularly useful when dealing with vector quantities such as forces 8 min read
• Vector Addition Vector Addition in Mathematics is the fundamental operation of vector algebra that is used to find the sum of two vectors. Vectors are mathematical quantities that have magnitude and direction. A vector can be represented by a line with an arrow pointing towards its direction and its length represen 15 min read
• Projectile Motion Projectile motion refers to the curved path an object follows when it is thrown or projected into the air and moves under the influence of gravity. In this motion, the object experiences two independent motions: horizontal motion (along the x-axis) and vertical motion (along the y-axis). Projectile 15+ min read

Chapter 4 - LAWS OF MOTION

• Newton's Laws of Motion | Formula, Examples and Questions Laws of Motion are the foundation of classical mechanics in physics given by the famous English physicist Sir Isaac Newton. These laws describe the behavior of moving objects and how they interact with forces. What are Newton's Laws of Motion?Newton's Laws of Motion in physics are the fundamental la 11 min read
• Law of Inertia Law of Inertia is another name for the First Law of Motion given by Sir Isaac Newton. As Law of Inertia has been studied by various scholars, throughout the centuries, and it helped humanity to understand the various concepts of motion in a wide range of fields from aerospace to automobile design. T 11 min read
• Newton's First Law of Motion Newton’s First Law of Motion, also known as the law of inertia, states that a body always opposes its change in the state of motion. Newton's Laws of Motion were first proposed by Sir Isaac Newton in the late 17th century. Newton's First Law of Motion finds its importance in various other laws and i 15 min read
• Newton's Second Law of Motion: Definition, Formula, Derivation, and Applications Newton's Second Law of Motion is a fundamental principle that explains how the velocity of an object changes when it is subjected to an external force. This law is important in understanding the relationship between an object's mass, the force applied to it, and its acceleration. In this article, we 14 min read
• Newton's Third Law of Motion | Action & Reaction Newton's Third Law of Motion is one of the most fundamental principles in physics. It is also known as law of action and reaction. This law forms the basis of many interactions in our daily lives, from walking to the functioning of rockets. Newton's Third Law represents a specific symmetry in the na 15 min read
• Conservation of Momentum Assume a fast truck collides with a stopped automobile, causing the automobile to begin moving. What exactly is going on behind the scenes? In this case, as the truck's velocity drops, the automobile's velocity increases, and therefore the momentum lost by the truck is acquired by the automobile. Wh 12 min read
• Static Equilibrium Static Equilibrium refers to the physical state of an object when it is at rest and no external force or torque is applied to it. In Static Equilibrium, the word 'static' refers to the body being at rest and the word 'equilibrium' refers to the state where all opposing forces cancel out each other a 9 min read
• Types of Forces Forces are an external cause that makes a body move, stop, and increase its velocity and other. There are various types of forces in physics and they are generally classified into two categories that are, Contact Force and Non Contact Force. In general, we define a push and pull as a force, and forc 14 min read
• Friction Friction in Physics is defined as a type of force that always opposes the motion of the object on which it is applied. Suppose we kick a football and it rolls for some distance and eventually it stops after rolling for some time. This is because of the friction force between the ball and the ground. 8 min read
• Rolling Friction Rolling Friction is a frictional force that opposes rolling objects. Rolling friction is applicable where the body moves along its curved surfaces. For example, wheels in vehicles, ball bearings, etc. are examples of rolling friction. In this article, we will learn about rolling friction, its defini 10 min read
• Circular Motion Circular Motion is defined as the movement of an object rotating along a circular path. Objects in a circular motion can be performing either uniform or non-uniform circular motion. Motion of a car on a bank road, the motion of a bike, the well of death, etc. are examples of circular motion. In this 15+ min read
• Solving Problems in Mechanics One must have probably heard of Newton's Laws of Motion by now. These laws will assist you in addressing mechanical issues. Typically, a mechanics problem does not include numerous forces operating on a single item. On the contrary, it is concerned with an assembly of many bodies exerting forces on 10 min read

Chapter 5 - WORK, ENERGY AND POWER

• Energy Energy in Physics is defined as the capacity of a body to do work. It is the capacity to complete a work. Energy can be broadly categorized into two categories, Kinetic Energy and Potential Energy. The capacity of an object to do the work is called the Energy. In this article, we will learn about, E 11 min read
• Work Energy Theorem The concept "work" is commonly used in ordinary speech, and we understand that it refers to the act of accomplishing something. For example, you are currently improving your understanding of Physics by reading this article! However, Physics may disagree on this point. The Work-energy Theorem explain 12 min read
• Work - Definition, Formula, Types of Work, Sample Problems In daily life, you are doing activities like study, running speaking, hear, climbing, gossips with friends and a lot of other things. Do you know? All these activities require some energy, and you get it from your daily food. In our day-to-day life, everyone eats food, gets energy, and does some act 6 min read
• Kinetic Energy Kinetic Energy is the energy associated with an object moving with a velocity. For an object of mass m and velocity, its kinetic energy is half of the product of the mass of the object with the square of its velocity. In our daily life, we observe kinetic energy while walking, cycling, throwing a ba 10 min read
• Work Done by a Variable Force Usually, a dancing person is considered to be more energetic compared to a sitting person. A security guard who has been standing at his place the whole day has been working for hours. In real life, this seems obvious, but these terms and definitions work differently when it comes to physics. In phy 6 min read
• Potential Energy Potential energy in physics is the energy that an object possesses as a result of its position. The term Potential Energy was first introduced by a well-known physicist William Rankine, in the 19th century. Gravitational Potential Energy, the elastic potential energy of an elastic spring, and the el 8 min read
• Mechanical Energy Formula Mechanical Energy - When a force operates on an object to displace it, it is said that work is performed. Work entails the use of a force to shift an object. The object will gather energy after the job is completed on it. Mechanical energy is the amount of energy acquired by a working object. The me 7 min read
• Potential Energy of a Spring A spring is used in almost every mechanical aspect of our daily lives, from the shock absorbers of a car to a gas lighter in the kitchen. Spring is used because of their property to get deformed and come back to their natural state again. Whenever a spring is stretched or compressed, a force is expe 7 min read
• Power Power in Physics is defined as the time rate of the amount of energy converted or transferred. In the SI system (or International System of Units), Watt (W) is the unit of Power. Watt is equal to one joule per second. In earlier studies, power is sometimes called Activity. Power is a scalar quantity 9 min read
• Collision Theory Collision Theory says that when particles collide (strike) each other, a chemical reaction occurs. However, this is necessary but may not be a sufficient condition for the chemical reaction. The collision of molecules must be sufficient to produce the desired products following the chemical reaction 7 min read
• Collisions in Two Dimensions A Collision occurs when a powerful force strikes on two or more bodies in a relatively short period of time. Collision is a one-time occurrence. As a result of the collision, the involved particles' energy and momentum change. The collision may occur as a result of actual physical contact between th 9 min read

Chapter 6 - SYSTEMS OF PARTICLES AND ROTATIONAL MOTION

• Concepts of Rotational Motion Rotational motion refers to the movement of an object around a fixed axis. It is a complex concept that requires an understanding of several related concepts. Some of the important concepts related to rotational motion include angular displacement, angular velocity, angular acceleration, torque, the 10 min read
• Motion of a Rigid Body A rigid body is a solid body that has little to no deformation when a force is applied. When forces are applied to such bodies, they come to translational and rotational motion. These forces change the momentum of the system. Rigid bodies are found almost everywhere in real life, all the objects fou 7 min read
• Centre of Mass Centre of Mass is the point of anybody where all the mass of the body is concentrated. For the sake of convenience in Newtonian Physics, we take the body as the point object where all its mass is concentrated at the centre of mass of the body. The centre of mass of the body is a point that can be on 15+ min read
• Motion of Center of Mass Center of Mass is an important property of any rigid body system. Usually, these systems contain more than one particle. It becomes essential to analyze these systems as a whole. To perform calculations of mechanics, these bodies must be considered as a single-point mass. The Center of mass denotes 7 min read
• Linear Momentum of a System of Particles The mass (m) and velocity (v) of an item are used to calculate linear momentum. It is more difficult to halt an item with more momentum. p = m v is the formula for linear momentum. Conservation of momentum refers to the fact that the overall quantity of momentum never changes. Let's learn more about 8 min read
• Relation between Angular Velocity and Linear Velocity Motion is described as a change in position over a period of time. In terms of physics and mechanics, this is called velocity. It is defined as the change in position over a period. Rotational Motion is concerned with the bodies which are moving around a fixed axis. These bodies in rotation motion o 4 min read
• Angular Acceleration Angular acceleration is the change in angular speed per unit of time. It can also be defined as the rate of change of angular acceleration. It is represented by the Greek letter alpha (α). The SI unit for the measurement of, Angular Acceleration is radians per second squared (rad/s2). In this articl 6 min read
• Torque and Angular Momentum For a rigid body, motion is generally both rotational and translation. If the body is fixed at one point, the motion is usually rotational. It is known that force is needed to change the translatory state of the body and to provide it with linear acceleration. Torque and angular momentum are rotatio 7 min read
• Torque Torque is the effect of force when it is applied to an object containing a pivot point or the axis of rotation (the point at which an object rotates), which results in the form of rotational motion of the object. The Force causes objects to accelerate in the linear direction in which the force is ap 10 min read
• Angular Momentum Angular Momentum is a kinematic characteristic of a system with one or more point masses. Angular momentum is sometimes called Rotational Momentum or Moment of Momentum, which is the rotational equivalent of linear momentum. It is an important physical quantity as it is conserved for a closed system 10 min read
• Equilibrium of Bodies The laws of motion, which are the foundation of old-style mechanics, are three explanations that portray the connections between the forces following up on a body and its movement. They were first expressed by English physicist and mathematician Isaac Newton. The motion of an item is related to the 7 min read
• Moment of Inertia Moment of inertia is the property of a body in rotational motion. Moment of Inertia is the property of the rotational bodies which tends to oppose the change in rotational motion of the body. It is similar to the inertia of any body in translational motion. Mathematically, the Moment of Inertia is g 15+ min read
• Kinematics of Rotational Motion It is not difficult to notice the analogous nature of rotational motion and kinematic motion. The terms of angular velocity and angular acceleration remind us of linear velocity and acceleration. So, similar to the kinematic equation of motion. Equations of rotational motion can also be defined. Suc 6 min read
• Dynamics of Rotational Motion Rigid bodies can move both in translation and rotation. As a result, in such circumstances, both the linear and angular velocities must be examined. To make these difficulties easier to understand, it is needed to separately define the translational and rotational motions of the body. The dynamics o 9 min read
• Angular Momentum in Case of Rotation About a Fixed Axis When a rigid body rotates around a fixed axis, it is called rotational motion. Rotational motion can be seen almost everywhere in daily lives. From the wheels of a car to the hands of the clock. All these objects are making rotational motion around a fixed axis. Similar to linear motion, rotation mo 5 min read

Chapter 7 - GRAVITATION

• Gravitational Force Newton’s Law of Universal Gravitation is used to explain gravitational force. Gravitational Force is a type of Non-contact force, the gravitational force is a force in nature that is always attractive and conservative. Gravitational Force is defined as the force of attraction experienced by two or m 8 min read
• Kepler's Laws of Planetary Motion Kepler's law of planetary motion is the basic law that is used to define the motion of planets around the stars. These laws work in parallel with Newton's Law and Gravitation Law and are helpful in studying the motion of various planetary objects. Kepeler's law provides three basic laws which are, K 10 min read
• State the Universal Law of Gravitation The Universal Law of Gravitation, a cornerstone of classical physics, explains the gravitational attraction between masses. In this article, we are going to learn the statement of the universal law of gravitation. State the Universal Law of Gravitation The Universal Law of Gravitation is a fundament 2 min read
• What Is Gravitational Constant? Answer: The gravitational constant, denoted by G, is a fundamental physical constant that represents the strength of the gravitational force between two objects with mass.The gravitational constant is a fundamental constant in physics that plays a crucial role in the law of universal gravitation for 1 min read
• Acceleration due to Gravity Acceleration due to gravity (or acceleration of gravity) or gravity acceleration is the acceleration caused by the gravitational force of attraction of large bodies. As we know that the term acceleration is defined as the rate of change of velocity with respect to a given time. Scientists like Sir I 9 min read
• Factors affecting Acceleration due to Gravity Take something in your hand and toss it down. Its speed is zero when you free it from your grip. Its pace rises as it descends. It flies faster the longer it goes. This sounds like acceleration. Acceleration, on the other hand, implies more than just rising speed. Pick up the same object and throw i 11 min read
• Gravitational Potential Energy The energy possessed by objects due to changes in their position in a gravitational field is called Gravitational Potential Energy. It is the energy of the object due to the gravitational forces. The work done per unit mass to bring the body from infinity to a location inside the gravitational field 13 min read
• Escape Velocity Escape velocity as the name suggests, is the velocity required by an object to escape from the gravitational barrier of any celestial object. "What happens when you throw a stone upward in the air?" The stone comes back to the Earth's surface. If we throw the stone with a much higher force still it 7 min read
• Artificial Satellites When looked at the night sky many heavenly bodies like stars, moon, satellites, etc are observed in the sky. Satellites are small objects revolving or orbiting around a planet or on object larger than it. The most commonly observed and known satellite is the moon, the moon is the satellite of Earth, 8 min read
• Binding Energy of Satellites Humans learn early in life that all material items have a natural tendency to gravitate towards the earth. Anything thrown up falls to the ground, traveling uphill is much more exhausting than walking downhill, Rains from the clouds above fall to the ground, and there are several additional examples 10 min read

Chapter 8 - Mechanical Properties of Solids

• Stress and Strain Stress and Strain are the two terms in Physics that describe the forces causing the deformation of objects. Deformation is known as the change of the shape of an object by applications of force. The object experiences it due to external forces; for example, the forces might be like squeezing, squash 12 min read
• Hooke's Law Hooke's law provides a relation between the stress applied to any material and the strain observed by the material. This law was proposed by English scientist Robert Hooke. Let's learn about Hooke's law, its application, and others, in detail in this article. What is Hooke’s Law?According to Hooke's 10 min read
• Stress-Strain Curve Stress-Strain Curve is a very crucial concept in the study of material science and engineering. It describes the relationship between stress and the strain applied on an object. We know that stress is the applied force on the material, and strain, is the resulting change (deformation or elongation) 12 min read
• Modulus of Elasticity Modulus of Elasticity or Elastic Modulus is the measurement of resistance offered by a material against the deformation force acting on it. Modulus of Elasticity is also called Young's Modulus. It is given as the ratio of Stress to Strain. The unit of elastic modulus is megapascal or gigapascal Modu 12 min read
• Elastic Behavior of Materials Solids are made up of atoms based on their atomic elasticity (or molecules). They are surrounded by other atoms of the same kind, which are maintained in equilibrium by interatomic forces. When an external force is applied, these particles are displaced, causing the solid to deform. When the deformi 10 min read

Chapter 9 - Mechanical Properties of Fluids

• What is Pressure? Pressure is the force applied to the surface of an object per unit area over which that force is distributed. Various units are used to express pressure. Some of these derive from a unit of force divided by a unit of area; the SI unit of pressure, the pascal (Pa), for example, is one newton per squa 9 min read
• Streamline Flow The substance that can change its form under an external force is defined as fluid. Whenever an external force is applied to a fluid, it begins to flow. The study of fluids in motion is defined as fluid dynamics. Have you ever noticed a creek flowing beneath the bridge? When you see a streamline, wh 7 min read
• Bernoulli's Principle Bernoulli's Principle is a very important concept in Fluid Mechanics which is the study of fluids (like air and water) and their interaction with other fluids. Bernoulli's principle is also referred to as Bernoulli's Equation or Bernoulli Theorem. This principle was first stated by Daniel Bernoulli 15+ min read
• What is Viscosity? Viscosity is the measurement of the resistance of the flowing liquid. Let us learn more about viscosity with an example suppose we take two bowls, one bowl contains water and the other has honey in it, we drop the content of both bowls then we see that water flows much faster than honey which conclu 12 min read
• Surface Tension Surface tension is the ability of fluid surfaces to contract into the smallest possible surface area. Have you ever found that even after filling a glass full of water, you can only add a few more drops before it spills? Have you ever lost a thermometer and watched how the mercury reacts as it falls 11 min read

Chapter 10 - Thermal Properties of Matter

• Thermal Properties of Matter Thermal Properties of Matter refer to the characteristics and behaviors of substances related to heat and temperature. These properties play a crucial role in understanding how materials respond to changes in temperature and how they conduct store, or transfer heat. Some of the key thermal propertie 14 min read
• Difference between Heat and Temperature Heat and Temperature are two related terms that people may confuse often. It should be noted that Heat and temperature are two different quantities. The fundamental difference between heat and temperature is that Heat is the form of energy that transfers from a hot state to a cold state. The unit of 5 min read
• Temperature Scales Temperature is a physical parameter that indicates how hot or cold something is. It is required especially for the calculation of the average kinetic energy of the particles in an item. This is a form of energy that is related to movement. But how can someone tell how hot it is and how chilly it is? 9 min read
• Ideal Gas Law The ideal gas law also called the general gas equation, is an equation that provides the relation among the various parameters of the gas i.e. they provide the relation among pressure(P), temperature(T), and Volume(V) of the gas. It is a combination of Charles’s law, Boyle’s Law, Avogadro’s law, and 10 min read
• Thermal Expansion When it comes to liquids, it is observed that when a thermometer is placed in slightly warm water, the mercury in the thermometer rises. When we remove the thermometer from the heated water, the mercury level drops. Similarly, When a balloon is halfway inflated in a cool room and placed in warm wate 6 min read
• Specific Heat Capacity Specific Heat Capacity is one of the fundamental physical properties of matter that describes the amount of heat energy required to increase the temperature of 1 kg of matter by 1o Celsius. the SI unit of Specific Head Capacity is J/(KgK), but other than this Specific Heat Capacity is also measured 11 min read
• Calorimetry The Universe is made up of two elements: Matter and Energy. The matter is made up of atoms and molecules, and energy causes these atoms and molecules to move constantly – either by vibrating back and forth or colliding with one another. This movement of molecules and atoms generates a type of energy 14 min read
• Change of State of Matter When cubes of ice melt into water or liquid boils into vapor, you may have seen changes in states of matter, but have you ever wondered why the substances change their form? When matter loses or gains energy, it changes its condition. When a substance gains energy, its molecules or atoms move faster 6 min read
• Heat Transfer Formulas Heat is a measure of thermal energy that can be transferred from one point to another. Heat is the transfer of kinetic energy from an energy source to a medium or from one medium or object to another medium or object. Heat is one of the important components of phase changes associated with work and 6 min read
• Newton's Law of Cooling Newton's Law of Cooling is the fundamental law that describes the rate of heat transfer by a body to its surrounding through radiation. This law state that the rate at which the body radiate heats is directly proportional to the difference in the temperature of the body from its surrounding, given t 9 min read

Chapter 11 - Thermodynamics

• Thermodynamics Thermodynamics is a branch of Physics that explains how thermal energy is changed to other forms of energy and the significance of thermal energy in matter. The behavior of heat, work, and temperature, along with their relations to energy and entropy are governed by the Four Laws of Thermodynamics. 15+ min read
• Basics Concepts of Thermodynamics Thermodynamics is concerned with the ideas of heat and temperature, as well as the exchange of heat and other forms of energy. The branch of science that is known as thermodynamics is related to the study of various kinds of energy and its interconversion. The behaviour of these quantities is govern 12 min read
• Zeroth Law of Thermodynamics Zeroth Law of Thermodynamics states that when two bodies are in thermal equilibrium with another third body than the two bodies are also in thermal equilibrium with each other. Ralph H. Fowler developed this law in the 1930s, many years after the first, second, and third laws of thermodynamics had a 7 min read
• Heat, Internal Energy and Work Have ever wondered how does a heat engine work? or what's going in a glass of water kept on the table? When we normally observe a steady glass of water no kinetic or mechanical energy is noticed. But, when noticed under a microscope rapid motion of molecules is observed which determines the internal 8 min read
• First Law of Thermodynamics First Law of Thermodynamics adaptation of the Law of Conservation of Energy differentiates between three types of energy transfer: Heat, Thermodynamic Work, and Energy associated with matter transfer. It also relates each type of energy transfer to a property of a body's Internal Energy. The First L 8 min read
• Thermodynamic State Variables and Equation of State The branch of thermodynamics deals with the process of heat exchange by the gas or the temperature of the system of the gas. This branch also deals with the flow of heat from one part of the system to another part of the system. For systems that are present in the real world, there are some paramete 5 min read
• Thermodynamic Processes Thermodynamics has become an integral element of our daily lives. Whether you're in a car, sitting comfortably in an air-conditioned room, or sipping a cold beverage from the refrigerator, thermodynamics is used practically everywhere, either directly or indirectly. When "Sadi Carnot" the father of 10 min read
• Second Law of Thermodynamics Second Law of Thermodynamics defines that heat cannot move from a reservoir of lower temperature to a reservoir of higher temperature in a cyclic process. The second law of thermodynamics deals with transferring heat naturally from a hotter body to a colder body. Second Law of Thermodynamics is one 10 min read
• Reversible and Irreversible Processes The thermodynamics is the science that meant to study the energy transitions into heat and mechanical work, and flowing the energy from point to another point. It illustrates the reasons behind of many processes in nature such as fusion, freezing, evaporation, and sublimation processes, which also i 4 min read
• Carnot Engine A Carnot motor is a hypothetical motor that works on the Carnot cycle. Nicolas Leonard Sadi Carnot fostered the fundamental model for this motor in 1824. In this unmistakable article, you will find out about the Carnot cycle and Carnot Theorem exhaustively. The Carnot motor is a hypothetical thermod 5 min read

Chapter 12 - Kinetic Theory

• Kinetic Theory of Matter Kinetic Theory of Matter states that "All matter is made up of microscopic particles in random motion with space between them." All the objects around us are called matter and there are various phases of matter. The most common phase of the matter is, Solid, Liquid, and Gas. And energy of the partic 9 min read
• Molecular Nature of Matter - Definition, States, Types, Examples The distinct forms that different phases of matter take on is called the state of matter. The most common state matter that is easily observable in daily life is - Solid, liquid, gas and plasma. There are many other states known to us like - Bose-Einstein condensate and neutron degenerate matter, bu 9 min read
• Behavior of Gas Molecules - Kinetic Theory, Boyle's Law, Charles's Law The kinetic theory of gases is a simple, historically significant classical model of gas thermodynamic behavior that laid the groundwork for many fundamental thermodynamic notions. A gas is described by the model as a vast number of identical submicroscopic particles (atoms or molecules) moving in a 9 min read
• Kinetic Theory of Gases Kinetic Theory of Gases is a theoretical model which helps us understand the behavior of gases and their constituent particles. This theory suggests that gas is made up of a larger number of tiny particles which collide with each other and their surroundings and exchange kinetic energy between them. 11 min read
• Law of Equipartition of Energy Law of Equipartition of Energy has many names such as Equipartition Theorem, Equipartition Principle, Law of Equipartition, or simply Equipartition and it describes the distribution of energy among the particles in a system that is at thermal equilibrium. The law of Equipartition of Energy tells us 10 min read
• Mean Free Path - Definition, Formula, Derivation, Examples The kinetic theory was introduced to explain the structure and composition of molecules with respect to submicroscopic particles. The theory talks about the increase in pressure due to the constant movement and collision of the submicroscopic particles. It also discusses other properties of a gas su 6 min read

Chapter 13 - Oscillations

• Oscillation Oscillations are defined as the process of repeating vibrations of any quantity about its equilibrium position. The word “oscillation” originates from the Latin verb, which means to swing. An object oscillates whenever a force pushes or pulls it back toward its central point after displacement. This 8 min read
• Oscillatory and Periodic Motion There are many types of motions that are encountered in daily life- rectilinear motion and the motion of a projectile is one such example. Both of these motions are non-repetitive. It means that the object does not come back to the same place again. There are some motions that are repetitive in natu 7 min read
• Simple Harmonic Motion Simple Harmonic Motion is a fundament concept in the study of motion, especially oscillatory motion; which helps us understand many physical phenomena around like how strings produce pleasing sounds in a musical instrument such as the sitar, guitar, violin, etc., and also, how vibrations in the memb 15+ min read
• Uniform Circular Motion Uniform Circular Motion as the name suggests, is the motion of a moving object with constant speed in a circular path. As we know, motion in a plane only has two coordinates, either x, and y, y and z, or z and x. Except for Projectile motion, circular motion is also an example of motion in a 2-D pla 9 min read
• Velocity and Acceleration in Simple Harmonic Motion Simple Harmonic Motion is a periodic motion that repeats itself after a certain time period. It can be seen almost everywhere in real life, for example, a body connected to spring is doing simple harmonic motion. It is essential to know the equation for the position, velocity, and acceleration of th 5 min read
• Force Law for Simple Harmonic Motion Have you ever wondered why, when we stretch an elastic band and then let it go, it returns to its previous state? It is compelled to revert to its original state by a force. But what exactly is this force? Let us investigate this force and develop the force law for simple harmonic motion.  Periodic 15+ min read
• Energy in Simple Harmonic Motion Each and every object possesses energy. In a simple harmonic motion, the object goes to the extreme and acquires potential energy. When the object comes back to the mean position, its velocity is at its maximum. Thus, in this case, the potential is converted to kinetic energy and vice versa. In an i 5 min read

Chapter 14 - Waves

• Introduction to Waves - Definition, Types, Properties A wave is a propagating dynamic disturbance (change from equilibrium) of one or more quantities in physics, mathematics, and related subjects, commonly described by a wave equation. At least two field quantities in the wave medium are involved in physical waves. Periodic waves occur when variables o 11 min read
• Difference Between Longitudinal and Transverse Waves Difference Between Longitudinal and Transverse Waves: Waves are disturbance that travels in the medium and transfer energy. Waves are of different types: mechanical, electromagnetic, and matter waves. The longitudinal waves are waves in which particles present in the medium travel parallel along wit 5 min read
• Speed of a Travelling Wave In general, almost every material and object on earth has elastic binding forces. When the body is compressed or released, these elastic forces start acting and the motion of one part of the system affects other parts. Thus, the motion of one part of the system affects the other parts of the system 5 min read
• Principle of Superposition of Waves When two waves propagating in the same medium interfere with each other the amplitude of the resultant of the two waves is the vector sum of the amplitude of the two waves, this is called the Principle of Superposition of Waves. Waves are disturbances that transfer energy between two points without 10 min read
• Reflection of Waves Waves are the disturbance created in the surroundings which are used to transport energy from point A to point B without transfer of matter. We also see different types of waves in our surroundings, when we throw a stone in the quiet pond we observe a disturbance travelling in the pond water formed 10 min read
• Beat Frequency Formula Sound waves are referred to as the beat. The difference in frequency between two waves is called the beat frequency. Interference, both helpful and detrimental, is to blame. In sound, the beat frequency is defined as the rate at which the loudness of the sound fluctuates, whereas the ordinary freque 2 min read
• School Learning
• School Physics
• Physics-Class-9

Improve your Coding Skills with Practice

What kind of Experience do you want to share?

• Kinetic Energy

If we need to quicken an item, at that point we should apply a power. Applying a power expects us to accomplish work. After work has been done, energy has been moved to an object. The item will be moving with another consistent speed. The energy moved is kinetic energy. It relies upon the mass and speed accomplished.

What is Kinetic Energy?

In science, the kinetic energy (KE) of an object is the energy that it has because of its movement. It is the work that is expected to quicken a body of a given mass from rest to its expressed speed. While picking up the energy during its quickening process.

The body keeps up this kinetic energy except if its speed changes. A similar measure of work that is finished by the body while it is decelerating from its present speed to a condition of rest. In traditional mechanics, the kinetic energy of a non-turning object of mass m going at a speed v is \(\frac{1}{2}mv^{2}\). In relativistic mechanics, v is significantly less than the speed of light.

The standard unit of kinetic energy is the Joule. While the supreme unit of kinetic energy is the foot-pound.

History of Kinetic Energy

The kinetic energy has its underlying foundations in the Greek word kinesis, signifying “movement”. The division between kinetic energy and potential energy can be followed back to Aristotle’s ideas.

The rule in traditional mechanics that E =\(mv^{2}\) was first evolved by Gottfried Leibniz and Johann Bernoulli. He depicted kinetic energy as living power. The Willem’s Gravesande belonging to the Netherlands gave trial pGravesande established that their entrance profundity was relative to the square of their effect speed.roof of this relationship. Following the process of dropping loads from various statures into a square of mud. Willem’s

The terms kinetic energy and work in their implications go back to the mid-nineteenth century. Early understandings of these thoughts can be ascribed to Gaspard-Gustave Coriolis. He, in 1829 distributed the paper named Du Calcul de l’Effet des Machines illustrating the arithmetic of active energy. William Thomson, later Lord Kelvin, is given the acknowledgement for begetting the expression “kinetic energy”.

Calculation of Kinetic Energy

To calculate kinetic energy, we follow the outline and begin by finding the work done,  W , by a force ,  F . Consider a box of mass  m  being pushed through a distance  d  along a surface by a force parallel to that surface. As we know,

\(\therefore\) F= m.a.d

We know kinematic equations of motion. If we substitute the acceleration in the equation.

Rearranging the kinematic formula \(v^2\) =\(v_0^2+2ad\) gives us a=\(\dfrac{v^2-v_{0}^2}{2d}\)

The initial and final velocity is \(v_\mathrm{i}\) and \(v_\mathrm{f}\)

So,  W = \(m\cdot d\cdot \frac{v_\mathrm{f}^2-v_\mathrm{i}^2}{2d}\)

= \(m\cdot \frac{v_\mathrm{f}^2-v_\mathrm{i}^2}{2}\)

= \(\frac{1}{2}\cdot m \cdot v_\mathrm{f}^2 – \frac{1}{2}\cdot m \cdot v_\mathrm{i}^2\)

So, the net amount of kinetic energy is \(\frac{1}{2}mv^2\)

Alternatively, the change in kinetic energy is equal to the net work done on an object or system.

\(W_{net}\)=\(\Delta K\)

This conclusion is the work-energy theorem. It applies quite generally, even with forces that vary in direction and magnitude.

The Kinetic Energy of Systems

An arrangement of bodies may have inner kinetic energy. This is because of the overall movement of the bodies in the framework. For instance, in a tank of gas, the particles are moving every which way. The kinetic energy of the framework is the amount of the active energies of the bodies it contains.

A visible body that is fixed. For example, a reference outline has been decided to relate to the body’s focal point of force may have different sorts of inward energy. It can be at the sub-atomic or nuclear level, which might be viewed as kinetic energy, because of sub-atomic interpretation, turn, and vibration, electron interpretation and turn, and atomic turn. These all add to the weight’s, as given by the uncommon hypothesis of relativity. While talking about developments of a perceptible body, the active energy alluded to is normally that of the naturally visible development as it were. However, all inward energies of different types add to weight’s, latency, and absolute energy.

Types of Kinetic Energy

There are five kinds of kinetic energy that are brilliant, warm, solid, electrical and mechanical.

Radiant Energy

Radiant energy is a sort of kinetic energy as it is consistently moving to go through medium or space. The energy is made through electromagnetic waves and is most regularly experienced by people as warmth. Instances of brilliant energy are:

• Ultraviolet light
• Gamma beams

Thermal Energy

Thermal energy or heat energy is produced because of the fast movement of iotas when they slam into one another. Warm energy is like brilliant energy in that both can be knowledgeable about the type of warmth or warmth. The thing that matters is that while brilliant energy alludes to waves or particles. Thermal energy depicts the degree of action among the iotas and atoms in an item. Instances of warm energy are:

• Hot springs
• Heated pool

Sound Energy

Sound energy is created by the vibration of an object. It goes through the medium yet can’t go in a vacuum as there are no particles to go about as a medium. Instances of sound energy are:

• Tuning fork
• Beating drums

Electrical Energy

Electrical energy is acquired from the free electrons that are of positive and negative charge. Instances of electrical energy are:

• Batteries at the time of use

Mechanical Energy

The amount of dynamic energy and potential energy is mechanical energy. One can neither create nor devastate it. However, we can change it over from one structure to others. Instances of mechanical energy are:

• Orbiting of satellites around the earth
• A moving vehicle

FAQs about Kinetic Energy

Q.1. Write a short note on kinetic energy?

Answer: Kinetic energy is the energy of an object has due to its movement.

On the off chance that we need to quicken an item, at that point we should apply a force. Applying a force expects us to accomplish work. After work has been done, energy has been moved to the item. The object will be moving with another steady speed. The energy moved is kinetic energy. It relies upon the mass and speed accomplished.  We can move kinetic energy among objects and change the kinetic energy into different sorts of energy. For instance, a flying squirrel may slam into a fixed chipmunk. Following the crash, a portion of the underlying dynamic energy of the squirrel may have been moved into the chipmunk or changed to some other type of energy.

Q.2. Can the kinetic energy be negative?

Answer: Kinetic energy can’t be negative, in spite of the fact that the change in dynamic energy \(\Delta K\), K can be negative. Since mass can’t be negative and the square of speed gives a non-negative number, kinetic energy can’t be negative. Either something is moving and has positive kinetic energy, or it isn’t moving and has zero dynamic energy. On the off chance that the last speed is not exactly the underlying rate, at that point the last active energy is not exactly the underlying dynamic energy and \(\Delta K\), K is negative.

Q.3. When is the kinetic energy maximum?

Answer: At the point when the kinetic energy is most extreme, the potential energy is zero. This happens when the speed is most extreme and the mass is at the harmony position. The potential energy is greatest when the speed is zero. The all-out energy is the amount of the kinetic energy in addition to the expected energy and it is consistent.

Customize your course in 30 seconds

Which class are you in.

Work and Energy

• Energy Level
• Work and Energy  
• Introduction to Work
• Energy and Types of Energy

Leave a Reply Cancel reply

Your email address will not be published. Required fields are marked *

Download the App